How Is Potential Energy Represented In Physics?
Definition of Potential Energy
Potential energy is stored energy due to an object’s position or chemical configuration. It represents the capacity to do work or produce change. An object can have potential energy by virtue of its height above the ground, its elastic deformation, its chemical composition, or how its parts are configured.
Potential energy is one of the two major types of mechanical energy, along with kinetic energy. Kinetic energy is energy of motion, whereas potential energy reflects stored energy due to an object’s structure or placement. Potential energy is a property, not a force, and depends on the physical system’s configuration.
Some key characteristics of potential energy:
- Stored energy that has the potential to be converted into other forms
- Scalar quantity, meaning it has magnitude but no direction
- Can take multiple forms – gravitational, elastic, chemical, nuclear
- In a closed system, total mechanical energy (potential + kinetic) is conserved
Types of Potential Energy
There are several main types of potential energy that are important in physics:
Gravitational Potential Energy
Gravitational potential energy is energy stored in an object due to its height above the ground. The higher the object is above the ground, the greater its gravitational potential energy. This energy gets converted to kinetic energy as the object falls.
Elastic Potential Energy
Elastic potential energy is energy stored in elastic materials that are stretched or compressed. For example, a stretched rubber band has elastic potential energy that can do work when released.
Chemical Potential Energy
Chemical potential energy is energy stored in the chemical bonds of substances. This energy can be released through chemical reactions. Examples include the energy stored in food, fuel, and batteries.
Nuclear Potential Energy
Nuclear potential energy is energy stored in the nucleus of an atom due to forces between nucleons. This energy can be released in nuclear fission or fusion reactions.

Gravitational Potential Energy
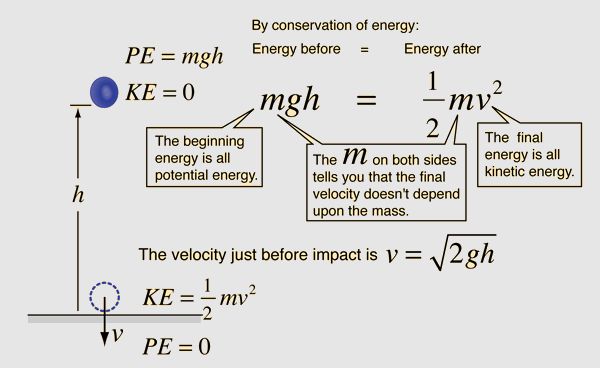
Gravitational potential energy depends on the height of an object, its mass, and the strength of the gravitational field. The higher the object is above the ground, the greater its gravitational potential energy. More massive objects also have greater gravitational potential energy at a given height. Additionally, gravitational potential energy is directly proportional to the strength of the gravitational field. The gravitational field strength on Earth is determined by the gravitational constant G. Increasing the mass of the Earth would increase the gravitational field strength and thus increase an object’s gravitational potential energy.
The formula for gravitational potential energy is:
PEgrav = mgh
Where m is the mass of the object, g is the gravitational field strength, and h is the height of the object.
Gravitational potential energy is converted to kinetic energy as an object falls. The sum of kinetic and potential energy remains constant, demonstrating the conservation of mechanical energy.
Elastic Potential Energy
Elastic potential energy depends on the spring constant of an elastic object like a spring or rubber band. It refers to the potential energy stored in an elastic object that is stretched or compressed. The spring constant (k) determines how stiff and resistant to deformation an elastic object is. The displacement (x) refers to how much an elastic object is stretched or compressed from its equilibrium or relaxed position.
The equation for elastic potential energy is:
PEelastic = 1/2 kx2
Where:
- PEelastic is the elastic potential energy (in Joules)
- k is the spring constant (in N/m)
- x is the displacement from equilibrium (in meters)
The more a spring or elastic object is stretched or compressed, the more potential energy it stores. This potential energy can be converted into kinetic energy if released, such as a slingshot firing or a compressed spring bouncing back.
Chemical Potential Energy
Chemical potential energy is the energy stored in the chemical bonds of atoms and molecules. It is the energy that can be released or absorbed during a chemical reaction. When a chemical reaction occurs, the chemical bonds between atoms are broken and rearranged to form new chemical bonds. This process results in a net release or absorption of energy.
For example, when fossil fuels like coal, oil and natural gas undergo combustion reactions with oxygen, the chemical bonds in the fuels are broken down and energy is released in the form of heat. This released energy can be used to produce mechanical work. The amount of chemical potential energy stored in a sample of fuel depends on the types of bonds present and the structure of the molecules. Substances with more complex, higher energy bonds tend to possess more potential energy.
Foods and batteries are other examples of substances rich in chemical potential energy. Foods contain high-energy biomolecules like carbohydrates, fats and proteins. Batteries derive their electrical energy from reduction-oxidation reactions which rearrange chemical bonds. In both cases, chemical potential energy is converted into other useful forms of energy.
The key takeaway is that chemical potential energy exists in the very structure of molecules. This energy can be tapped in chemical reactions and converted into thermal, mechanical or electrical energy. It is an essential source of energy for many biological and industrial processes.
Nuclear Potential Energy
Nuclear potential energy refers to the energy stored within an atom’s nucleus. It is the energy that holds the nucleus together. The nuclear force between the protons and neutrons overcomes the repulsive electric force between the positively charged protons.
Nuclear potential energy depends on the binding energy of the nucleus. Binding energy is the amount of energy needed to split the nucleus apart into individual protons and neutrons. The more tightly bound the nucleus, the more energy is required to break it apart, and therefore the greater the nuclear potential energy.
Nuclear potential energy can be released in two main ways: nuclear fission and nuclear fusion.
In nuclear fission, a large unstable nucleus splits into smaller nuclei, releasing energy in the process. For example, uranium or plutonium nuclei can undergo fission when bombarded with neutrons, producing lighter elements and radiation.
In nuclear fusion, light nuclei are fused together to create heavier nuclei, converting some mass into energy based on Einstein’s equation E=mc2. Fusion occurs at extremely high temperatures, like those present at the core of the Sun, where hydrogen nuclei fuse to form helium and release tremendous amounts of energy.
Both fission and fusion reactions harness the nuclear potential energy stored in atomic nuclei. This energy can be used to produce electricity, like in nuclear power plants, or in nuclear weapons.
Potential Energy Graphs
One way to visualize potential energy is through graphs showing the relationship between potential energy (PE) and position. These PE graphs illustrate how the PE of an object or system changes depending on location or configuration.
For conservative forces like gravity, the PE graphs show that energy is stored in objects when they are in higher positions. As the object moves to lower positions, this PE gets converted to kinetic energy. The graphs demonstrate how total mechanical energy (PE + KE) is conserved.
On PE graphs:
- The vertical axis represents PE
- The horizontal axis represents position
- The curve illustrates how PE changes with position
- Higher positions mean more stored PE
- As objects fall, PE decreases and KE increases
- PE graphs visualize the energy transfers and transformations in a system
PE graphs are useful for understanding concepts like energy conservation, stability, and equilibrium. They provide a mathematical visualization for potential energy in physics.
Conservation of Mechanical Energy
The principle of conservation of mechanical energy states that in an isolated system that is not subject to any external forces, the total mechanical energy remains constant. Mechanical energy refers to the sum of kinetic energy and potential energy in a system.
Kinetic energy is the energy associated with motion and depends on the mass and velocity of an object. Potential energy depends on the position or configuration of an object within a field of force such as gravity or magnetism. It is the stored energy an object has by virtue of its position.
According to the law of conservation of mechanical energy, the total initial mechanical energy of an isolated system equals the total final mechanical energy of the system. That is, the initial kinetic energy plus initial potential energy equals the final kinetic energy plus final potential energy.
This principle applies in situations where an object moves without any influence from external forces. The mechanical energy transforms back and forth between kinetic and potential, but the total amount of mechanical energy remains fixed.
For example, a pendulum swinging back and forth exchanges kinetic and gravitational potential energy during its motion, but the total mechanical energy at any point remains constant if we neglect frictional losses. The conservation of mechanical energy is a fundamental concept in physics with many applications in mechanical systems.
Potential Energy Examples
Potential energy can be illustrated through several common real-world examples:
Pendulum
A pendulum swinging back and forth demonstrates gravitational potential energy being converted into kinetic energy. At the highest point of the swing, the pendulum has maximum gravitational potential energy. As it swings downward, this potential energy is converted into kinetic energy, reaching maximum kinetic energy at the lowest point. The kinetic energy then converts back into gravitational potential energy as it swings upward again.
Water Dam
In a hydroelectric dam, water held behind the dam has potential energy. When the water is released to flow through turbines, the potential energy gets converted into electricity. The higher the dam, the greater the potential energy stored in the water.
Stretched Spring
A stretched or compressed spring illustrates elastic potential energy. The spring gains potential energy when it is stretched or compressed. If released, this elastic potential energy will convert into kinetic energy as the spring returns to its original shape.
Applications of Potential Energy
Potential energy has many useful applications in our everyday lives and modern technology:
Batteries
Batteries store chemical potential energy and convert it into electrical energy that powers countless devices. The chemical reactions inside a battery generate charged particles that can do work. Batteries allow energy to be portable and accessible.
Power Plants
Power plants like hydroelectric dams and nuclear plants rely on potential energy being converted into electricity. For dams, the gravitational potential energy of water powers turbines as it flows downhill. In nuclear plants, nuclear potential energy is released from atoms and used to heat water into steam that drives turbines. This generates the electricity that powers cities and towns.
Roller Coasters
Roller coasters utilize gravitational potential energy and kinetic energy. As a roller coaster cart goes down a steep drop, its potential energy is converted into kinetic energy, allowing it to reach high speeds. The kinetic energy is then converted back into potential energy as the cart travels up the next incline. This energy conversion allows the thrilling motions of roller coasters.