How To Find Potential Energy?
Define Potential Energy
Potential energy is stored energy that an object possesses due to its position or chemical composition. It is the energy an object has by virtue of its location or arrangement of parts. Potential energy can be thought of as stored energy waiting to be released.
For example, a ball held at a height above the ground contains gravitational potential energy due to the earth’s gravity exerting a downward force on the ball. If released, this potential energy will be converted into kinetic energy as gravity accelerates the ball downward. The higher the initial position, the greater the potential energy.
Other forms of potential energy include elastic potential energy stored in stretched or compressed springs, chemical potential energy stored in the bonds between atoms of a substance, and nuclear potential energy that holds atomic nuclei together against the electrical repulsion of protons. In each case, the potential energy exists as stored energy ready to be released under the right circumstances.
Types of Potential Energy
There are four main types of potential energy:
Gravitational Potential Energy
Gravitational potential energy depends on the position of an object in a gravitational field. It is the amount of energy stored in an object due to its height relative to the surface of the Earth or any other massive body. The higher the object, the greater its gravitational potential energy. Gravitational potential energy can be calculated using the mass of an object, acceleration due to gravity, and height of the object.
Elastic Potential Energy
Elastic potential energy refers to energy stored in elastic objects or materials that are deformed through the application of a force. Elastic potential energy results from the stretching or compressing of a spring, rubber band, or other elastic material. When released, the elastic material will spring back to its original shape, releasing the stored elastic potential energy.
Chemical Potential Energy
Chemical potential energy is energy stored in the bonds between atoms and molecules. Chemical potential energy can be released or absorbed during a chemical reaction when chemical bonds are broken or formed. Examples of stored chemical potential energy include batteries, gasoline, and food. The chemical energy stored in molecules can be converted to kinetic energy, electricity, or heat.
Nuclear Potential Energy
Nuclear potential energy is the energy stored within an atom’s nucleus. It originates from the strong nuclear force which binds protons and neutrons together. Nuclear potential energy can be released when the nucleus splits apart (fission) or fused together (fusion). Nuclear reactions convert very small amounts of matter into energy, as described by Einstein’s famous equation E=mc2. Nuclear power plants capture the nuclear potential energy in uranium or plutonium and convert it to electricity.
Calculating Gravitational Potential Energy
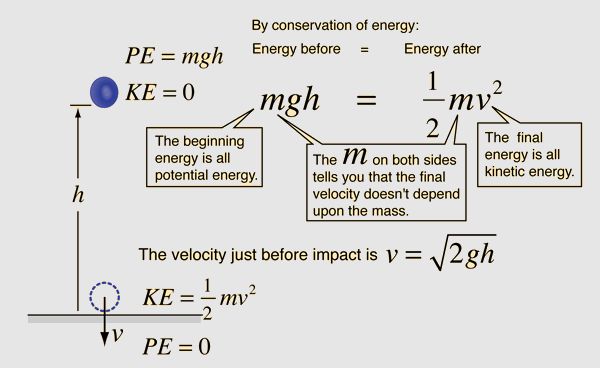
Gravitational potential energy depends on an object’s mass, the acceleration due to gravity, and the object’s height above a reference point. The formula for gravitational potential energy is:
PEgrav = mgh
Where:
- PEgrav is the gravitational potential energy in joules (J)
- m is the mass of the object in kilograms (kg)
- g is the acceleration due to gravity, which on Earth is 9.8 m/s2
- h is the height of the object above a reference point in meters (m)
Gravitational potential energy increases as the object is raised higher above the reference point. When the object falls, its potential energy is converted to kinetic energy. Gravitational potential energy can be harnessed using hydropower from dams or tidal power from coastal inlets.
Calculating Elastic Potential Energy
Elastic potential energy depends on the spring constant (k) of a spring or other elastic material and the amount of displacement (x) from the spring’s rest position. The formula for elastic potential energy is:
Elastic Potential Energy = 1/2 kx2
Where:
- k is the spring constant measured in N/m (Newtons per meter)
- x is the displacement from equilibrium in meters (m)
The spring constant (k) depends on the stiffness of the spring material. The stiffer the spring, the higher the spring constant. Displacement (x) refers to how much the spring is stretched or compressed from its resting position.
So the more a spring is displaced and the stiffer it is, the higher the elastic potential energy stored in the spring. This potential energy can be converted to kinetic energy if the spring is allowed to move from the displaced position back to equilibrium.
Calculating Chemical Potential Energy
Chemical potential energy depends on the types of molecular bonds within a substance. Some bonds require more energy to break than others, and thus have higher potential energy stored within them. The chemical potential energy can be calculated using the following steps:
1. Identify the types of chemical bonds present in the substance. Typical bonds include covalent, ionic, hydrogen bonds, and van der Waals forces.
2. Determine the bond energy values for each type of bond, which indicate the amount of energy required to break that bond. Bond energies are often measured in units of kilojoules per mole (kJ/mol).
3. Multiply the number of moles of each bond type by the corresponding bond energy. This provides the total potential energy stored in the chemical bonds.
4. Add together the potential energy contributions from each bond type to calculate the overall chemical potential energy.
The resulting number indicates how much energy is stored in the substance’s molecular structure. This energy could be released if chemical reactions break and form new bonds between atoms.
Calculating Nuclear Potential Energy
Nuclear potential energy depends on the binding energy within an atomic nucleus. Binding energy is the energy required to keep the protons and neutrons of an atom’s nucleus together. The more tightly bound the protons and neutrons are within the nucleus, the lower the potential energy. This binding energy acts against forces that try to separate the nucleus.
To calculate the nuclear potential energy of an atom, you need to know its atomic mass. The atomic mass can be used along with Einstein’s mass-energy equivalence equation (E=mc2) to determine the binding energy and thus the nuclear potential energy.
For example, the atomic mass of carbon-12 is 12 atomic mass units (amu). The mass of one atomic mass unit is 1.660 x 10-27 kg. Multiplying the atomic mass of carbon-12 by the mass of one amu gives the mass of a carbon-12 atom. This mass in kg can then be plugged into the mass-energy equivalence equation to find the binding energy and nuclear potential energy.
Real World Examples of Potential Energy
Potential energy is all around us in the real world. Here are some common real-world examples:
Boulder at the Top of a Hill
A large boulder sitting at the top of a hill contains gravitational potential energy. The higher it sits and the greater its mass, the more potential energy it holds. When the boulder rolls down the hill, that potential energy gets converted into kinetic energy.
Drawn Bow
When you draw back the string on a bow, you store elastic potential energy. As you pull the string farther, you increase the potential energy. When you release the string, the stored elastic potential energy gets converted into kinetic energy as the arrow flies through the air.
Batteries
Batteries contain stored chemical potential energy from the separation of positive and negative charges. When you connect a circuit, the chemical reactions inside the battery release the stored energy to power electrical devices.
Nuclear Reactors
Nuclear reactors contain enormous amounts of nuclear potential energy locked inside atomic nuclei. Nuclear fission reactions split the nuclei and release that nuclear energy, which gets converted to heat and used to generate electricity.
Converting Potential to Kinetic Energy
Potential energy can be converted into kinetic energy when an object that has stored potential energy starts moving. The potential energy gets used up in order to accelerate the object. This conversion occurs according to the Work-Energy theorem, which states that the net work done on an object equals its change in kinetic energy.
For example, imagine holding a book in your hand above the floor. The book has gravitational potential energy due to its height above the floor. If you let go of the book, gravity will accelerate it downwards and convert its potential energy into kinetic energy of motion. As it falls, the book’s potential energy decreases while its kinetic energy increases until it hits the floor.
The amount of potential energy that gets converted into kinetic energy depends on the mass of the object, its initial height, and any dissipative forces like air resistance that slow it down. Heavier objects or those dropped from greater heights will convert more potential energy and thus gain more kinetic energy and speed.
This conversion process demonstrates how energy can change forms yet follow the law of conservation of energy. The total initial potential energy does not disappear but is transformed into an equal amount of kinetic energy if we ignore nonconservative forces.
Potential Energy Diagrams
Potential energy diagrams, also known as potential energy curves, are a graphical representation of the potential energy of a system at different configurations. They illustrate how the potential energy changes as a function of position.
For example, in a simple spring system, the potential energy diagram would show the elastic potential energy stored in the spring at different amounts of compression or extension. At the natural, unstretched length of the spring, the potential energy would be at a minimum. As the spring is compressed or stretched away from the equilibrium position, the potential energy increases.
Potential energy diagrams are useful for visualizing energy transformations between potential and kinetic energy. The height of the curve corresponds to the amount of potential energy, while the slope of the curve represents the force. Steeper slopes mean greater forces.
These diagrams are commonly used in physics, chemistry, and engineering when analyzing things like molecular bonds, particle collisions, orbits, and more. They allow one to quickly identify the stable and unstable equilibrium states of a system.
Importance and Applications
Potential energy plays a crucial role in many areas of science and technology. Here are some of the key applications and importance of understanding potential energy:
Energy Storage and Transfer
Potential energy allows us to store energy for later use. For example, batteries store chemical potential energy, dams store gravitational potential energy in elevated water, and springs or elastic bands store elastic potential energy. This stored energy can then be transferred and converted to kinetic energy to do work.
Technology
Potential energy enables many modern technologies. Batteries that store chemical potential energy power our phones, laptops and electric cars. Hydroelectric dams use gravitational potential energy to generate electricity. Nuclear power plants convert nuclear potential energy to electrical energy. Understanding potential energy allows us to harness it effectively.
Safety
Knowing about potential energy helps identify and manage risks. Workers must be aware of gravitational potential energy when working at heights. Chemical engineers handle substances with chemical potential energy safely. Nuclear safeguards exist to control nuclear potential energy. By appreciating where potential energy exists, we can prevent dangerous releases of energy.