What Does Energy Per Charge Mean?

Energy per charge is a fundamental concept in physics that describes the amount of energy stored or released per unit of electric charge. It is commonly represented by the variables epsilon (ε) or U and has units of joules per coulomb (J/C). Understanding energy per charge provides key insights into how electric potential, voltage, electric fields, and electrostatic interactions work on a microscopic scale.

On an atomic level, energy per charge governs how electrons arrange themselves around nuclei and how atoms form bonds. In macroscopic systems, knowing the energy per charge helps calculate the work required to move a charge and determine the voltage between two points in a circuit. This concept is applicable to a wide range of phenomena from the operation of batteries and capacitors to biological processes. Overall, energy per charge is a foundational quantity in electrostatics critical for analyzing electrical components and systems.

Table of Contents

Units of Energy per Charge

Energy per charge is typically measured in units of electronvolts (eV) or joules per coulomb (J/C). An electronvolt is the amount of energy gained or lost by a single electron accelerating through an electric potential difference of 1 volt. It is a commonly used unit in atomic and nuclear physics. The electronvolt is defined as follows:

1 eV = 1.602 × 10−19 Joules

This means that 1 eV is equal to the amount of energy gained by one electron accelerating across a potential difference of 1 volt. The electronvolt provides a convenient scale for measuring atomic phenomena.

Joules per coulomb (J/C) is the SI unit for energy per charge. It equates to the amount of energy in joules that is gained or lost when 1 coulomb of charge moves between two points with a potential difference of 1 volt. The relationship between electronvolts and joules per coulomb is:

1 eV = 1.602 × 10−19 J/C

So an energy amount in electronvolts can easily be converted to joules per coulomb by multiplying by this conversion factor. Both units are commonly used when talking about energy levels, work functions, and other concepts in atomic and solid state physics.

Relation to Electric Potential

Energy per charge is closely related to the concept of electric potential in physics. Electric potential, also called voltage, describes the potential energy stored per unit charge at a location in an electric field. It is measured in units of joules per coulomb (J/C).

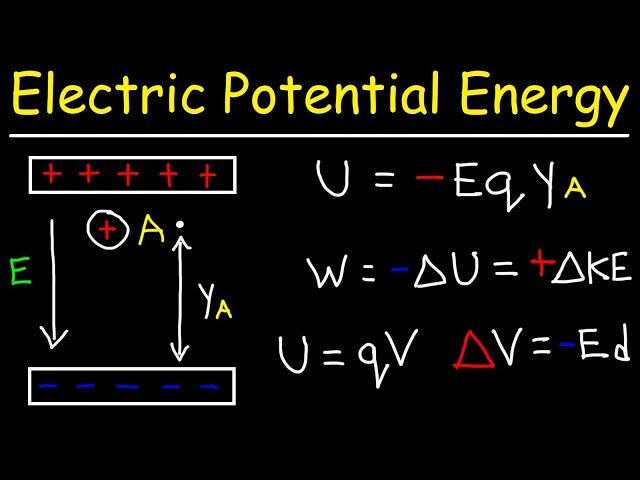
Electric potential causes positive charges to move from areas of low potential to high potential, while negative charges flow from high to low potential. So the electric potential difference between two points determines the direction and magnitude of charge flow. When charges move in an electric field, they gain or lose energy equal to the charge multiplied by the change in electric potential.

Since electric potential energy per charge equals voltage, energy per charge and electric potential are numerically equal. But conceptually they refer to distinct properties of the electric field. Energy per charge describes the energy stored in the field, while electric potential describes the forces acting on charges.

Calculating Energy per Charge

The energy per charge is calculated using the simple formula:

E = qV

Where E is the energy in joules (J), q is the electric charge in coulombs (C), and V is the electric potential difference or voltage in volts (V). This formula shows that the energy gained or lost by a charge q as it moves through a potential difference V is directly proportional to the amount of charge and the potential difference.

To calculate the energy per charge, you simply divide the total energy E by the amount of charge q. This gives the energy in joules per coulomb of charge, which is equivalent to volts.

For example, if a 5 C charge moves through a potential difference of 12 V, gaining 60 J of energy, the energy per charge would be:

E/q = 60 J / 5 C = 12 V

So in this case the energy per charge is numerically equal to the potential difference. This demonstrates that the volt unit is actually a derived unit, equal to joules per coulomb.

Energy Levels in Atoms

Electrons in atoms exist at discrete, quantified energy levels. The lowest energy level is called the ground state. Higher energy levels are called excited states. Electrons can move between different energy levels by gaining or losing energy.

The exact energy levels depend on the atom. They are determined by solutions to the Schrödinger equation for the atom’s potential. Each energy level is characterized by a distinct amount of energy per electron. The difference in energy between levels corresponds to definite amounts or quanta of energy an electron in the atom can possess.

When an electron transitions between levels, it emits or absorbs a photon of electromagnetic radiation equal to the exact energy difference between levels. The emission or absorption spectra of atoms provide a means to measure their quantized energy levels. Energy per charge quantifies the distinction between these discrete electron energy levels within atoms.

Applications

Energy per charge has important practical applications in various technologies and devices:

Batteries and Fuel Cells

In electrochemical cells like batteries and fuel cells, the voltage (electrical potential energy per charge) determines the amount of electrical energy that can be extracted. The higher the voltage, the more energy can be delivered per electron transferred through the external circuit.

Solar Cells

Solar cells absorb photons and generate electron-hole pairs with energy equal to the photon energy. The built-in electric field causes charge separation, resulting in voltage that drives current through an external load. The voltage is proportional to the energy per charge.

Electrolysis

Electrolysis uses electrical energy to drive a chemical reaction that would not occur spontaneously. The minimum voltage needed is proportional to the energy required to remove an electron from the species being oxidized at the anode. A higher voltage can deliver more energy per electron to drive the reaction.

Energy Bands in Solids

In solids, the discrete energy levels of individual atoms combine and split into bands of closely spaced energy levels. This is due to the close proximity of the atoms in a solid, which allows their electrons to interact and form bands.

The presence and properties of these energy bands have major implications for whether a material acts as a conductor, semiconductor, or insulator:

In conductors like metals, the bands are partially filled, allowing electrons to move freely.
In semiconductors, there is a small band gap between a filled valence band and an empty conduction band. Electrons can jump the band gap with added energy.

In insulators, a large band gap separates the bands, preventing electron flow.

Understanding energy bands is thus key to designing solid-state electronic devices and computing the electrical properties of materials.

Quantum Physics

One of the most important discoveries related to energy per charge is the quantization of energy at the atomic and subatomic level. In the early 20th century, scientists such as Max Planck and Albert Einstein realized that energy can only exist in discrete packets or “quanta”. This new quantum theory revolutionized our understanding of physics.

One example demonstrating quantized energy levels is the photoelectric effect. When light shines on a metal surface, electrons are emitted. However, Einstein found that below a certain frequency threshold, no electrons are emitted regardless of light intensity. This is because the photon energy is too low to dislodge electrons from their quantized energy levels in the metal. Only above the threshold frequency do photons carry enough energy per charge (each photon having an energy proportional to its frequency) to eject electrons.

The photoelectric effect was key evidence for light having both wave and particle properties. The frequency dependence revealed the particle nature of light, with energy quantized in photons. Yet phenomena like interference showed light also behaving as waves. This wave-particle duality applies to all quantum particles, which can exhibit properties of both waves and discrete particles.

Overall, the discovery of quantized energy levels in atoms and photons was pivotal to the development of quantum mechanics in the 20th century. It revealed that energy per charge is not continuous, but restricted to defined values at the microscopic scale. This quantization underlies many quantum effects central to our modern understanding of physics.

History

The concept of energy per charge has its origins in the 19th century studies of electricity and magnetism. Some key developments include:

Michael Faraday – In the 1830s, Michael Faraday conducted experiments showing that electric charge produced by chemical reactions depended on the amount of chemicals involved. This was an early quantitative measurement linking charge and energy.

James Clerk Maxwell – In the 1860s, Maxwell developed the theory of electromagnetism, unifying electricity, magnetism and light phenomena. His equations related the energy of an electromagnetic field to its electric potential.

J.J. Thomson – In 1897, Thomson showed that cathode rays were made of negatively charged particles, later called electrons. He measured the charge to mass ratio of electrons.

Robert Millikan – In 1910, Millikan published his experiment measuring the elementary electric charge on a single electron. This allowed the quantitative calculation of energy per unit charge.

These foundational experiments demonstrated that electric charge is quantized and can be related to energy levels. This paved the way for the modern concept of energy per charge used in quantum physics and electronics.

Conclusion

In summary, energy per charge is a fundamental concept in physics that measures the amount of energy stored or transported per unit charge. It is closely related to the electric potential, and can be calculated by determining the change in potential energy over a given amount of charge transfer. Understanding energy per charge is crucial in many areas of physics and engineering.

At the atomic level, energy per charge quantifies the discrete energy levels that electrons can occupy around nuclei. It allows us to model electronic behavior in atoms and molecules. In solids, the theory of energy bands explains how materials can be insulators, conductors, or semiconductors based on gaps between allowed energy levels. Energy per charge also enables many key technologies, from batteries and fuel cells to particle accelerators.

By examining the connections between potential energy, charge, and electric fields, the concept of energy per charge provides profound insight into the quantum nature of electricity and matter. It represents a fundamental bridge between the mechanics of charged particles and the energies associated with their configurations and motions. The ability to quantify and manipulate energy per charge has driven many pivotal discoveries in physics over the past two centuries.