How Do You Describe Motion In Science?

Introducing Motion

Motion is the act of changing position or moving from one place to another. The study of motion, also called kinematics, is a major branch of physics that describes the motion of objects and systems without considering the forces that cause motion. Understanding motion is central to understanding our universe.

Everything in the universe moves – from subatomic particles to galaxies. Studying motion allows us to predict the movement of objects using mathematics. This mathematical description of motion allows us to model real-world systems and make testable predictions.

By studying motion, physicists gain insight into the underlying laws that govern our universe. For example, Newton’s laws of motion form the foundation of classical mechanics and allow us to calculate the motion of objects on earth and in space. Overall, kinematics provides the framework to quantitatively analyze motion and is fundamental to many areas of physics and engineering.

Describing Position

To describe the position of an object, we need a reference frame or coordinate system. A reference frame provides a set of axes from which measurements can be made. Common reference frames include:

• Cartesian coordinate system – Uses perpendicular x, y, and z axes to pinpoint a location in space.
• Polar coordinate system – Uses a radial distance r and angle θ to locate a point in 2D space.
• Cylindrical coordinate system – Adds a z-axis to polar coordinates to locate a point in 3D space.
• Spherical coordinate system – Uses a radial distance r, polar angle θ, and azimuthal angle φ to pinpoint a location in 3D space.

The origin of the coordinate system serves as the reference point from which measurements are made. Positions are described relative to the origin using coordinates. Selecting an appropriate coordinate system simplifies analysis for the type of motion being described.

Displacement

Displacement is a vector quantity that refers to the change in position of an object. It specifies the position of an object with reference to a point, and tells us how far the object has moved from its original position and in which direction.

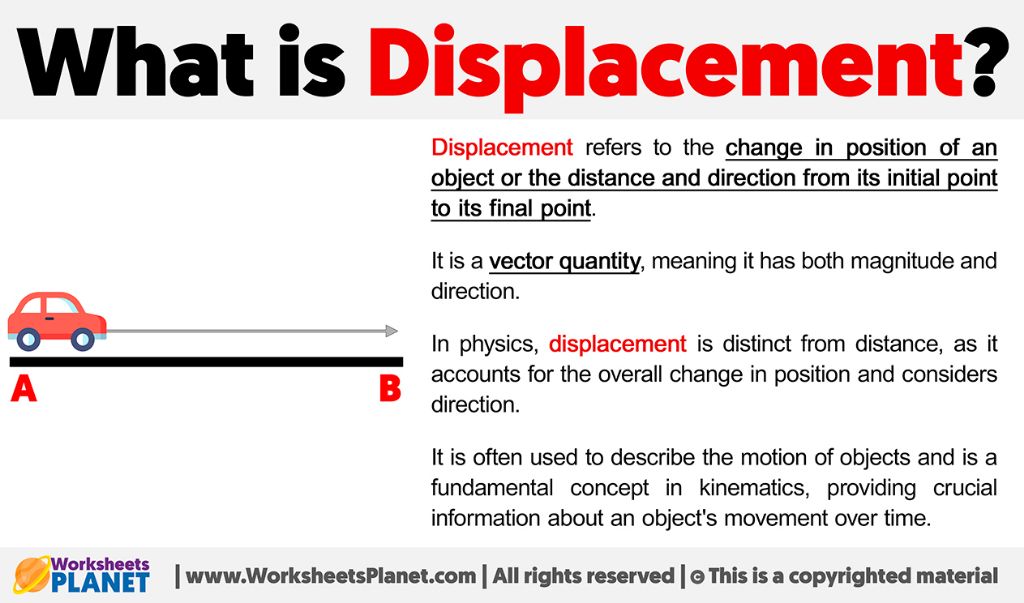
Displacement can be calculated as the difference between the final and initial positions of an object. It is typically represented by the symbol Δx. Mathematically, displacement = final position – initial position.

Displacement considers only the overall change in position, not the path traveled between the initial and final positions. An object that moves away from a point and then returns to the same point has zero displacement, even though it has moved.

The SI unit for displacement is the meter (m). Displacement may also be measured in centimeters (cm) or millimeters (mm). The direction of displacement is specified by a unit vector.

Examples of calculating displacement:

• If an object starts at position x = 5 m and moves to position x = 10 m, its displacement is Δx = 10 m – 5 m = 5 m.
• If a car begins at x = 2 km and ends at x = 6 km, its displacement is Δx = 6 km – 2 km = 4 km.

Displacement takes into account both distance and direction. Knowing an object’s displacement reveals how far it has moved from the origin as well as whether it moved to the left or right.

Velocity

Velocity describes the rate at which an object’s position changes over time. It refers to the speed of an object in a particular direction.

Definition

Velocity is defined as the rate of change of displacement with respect to time. It describes both the speed and direction of motion of an object.

Calculating Velocity

Velocity can be calculated by dividing the change in position (displacement) by the time taken. It is typically measured in meters per second (m/s) in metric units and miles per hour (mph) in imperial units.

Velocity = Displacement / Time

Or

v = Δd / Δt

Units

The SI unit for velocity is meters per second (m/s). Other common units include kilometers per hour (km/h), miles per hour (mph), and feet per second (ft/s).

Average vs Instantaneous Velocity

Average velocity refers to the total displacement divided by the total time over an interval. Instantaneous velocity is the velocity at a specific point in time.

Average velocity = Total displacement / Total time

Instantaneous velocity is the derivative of position with respect to time at a precise moment. It provides velocity at an exact point in the motion.

Acceleration

Acceleration describes how velocity changes over time. It refers to how quickly velocity increases or decreases, as well as the rate of change and direction.

Definition

Acceleration is defined as the rate of change of velocity with respect to time. It describes the rate at which velocity changes in terms of both magnitude and direction per unit time.

Calculating Acceleration

Acceleration can be calculated by finding the change in velocity (final velocity minus initial velocity) and dividing it by the time interval over which the change occurred.

The formula is:

Acceleration = (Final Velocity – Initial Velocity) / Time

or:

a = Δv / Δt

Units

The standard metric units for acceleration are meters per second squared (m/s2). This represents the change in velocity in meters per second over each second.

Average vs Instantaneous Acceleration

Average acceleration refers to the change in velocity over a time interval. Instantaneous acceleration is acceleration at a specific point in time.

Instantaneous acceleration provides the acceleration at that exact moment. Average acceleration provides the rate of change over the time interval.

Equations of Motion

An important set of equations for describing motion are the kinematic equations. Kinematics is the study of motion without considering forces. The kinematic equations describe the relationship between key quantities like displacement, velocity, acceleration and time.

There are four key kinematic equations:

v = u + at

This equation shows that velocity (v) equals the initial velocity (u) plus the acceleration (a) times time (t). It describes how velocity changes under constant acceleration.

s = ut + 1/2at^2

This equation shows that displacement (s) equals the product of initial velocity (u) and time (t) plus one half the acceleration (a) times time (t) squared. It describes the relationship between displacement, velocity, acceleration and time.

v^2 = u^2 + 2as

This equation shows that the final velocity (v) squared equals the initial velocity (u) squared plus 2 times the acceleration (a) times the displacement (s). It relates velocity, acceleration and displacement.

s = (v + u)t/2

This equation shows that displacement (s) equals the average of initial (u) and final velocity (v) multiplied by time (t). It describes displacement in terms of average velocity.

By using these fundamental equations, along with known values, we can analyze all kinds of motion problems and make predictions. They allow motion to be described mathematically in useful ways.

Graphical Analysis

Graphs are a useful tool for visualizing and analyzing motion. There are three common types of graphs used:

Position-Time Graphs

Position-time graphs show how an object’s position changes over time. The slope or steepness of the graph indicates the velocity. A steeper slope means faster velocity. The area under the graph corresponds to the object’s displacement.

Velocity-Time Graphs

Velocity-time graphs illustrate how an object’s velocity changes over time. The slope or steepness of the graph indicates the acceleration. A steeper slope means greater acceleration. The area under the graph corresponds to the object’s displacement.

Acceleration-Time Graphs

Acceleration-time graphs show how the acceleration of an object changes over time. The slope indicates the rate of change of acceleration, or jerk. These graphs help identify periods of speeding up, slowing down, or constant velocity.

Analyzing these three graphs together provides a detailed visualization of an object’s motion under different conditions.

Vector Nature

Motion can be described using either scalars or vectors. Scalars only have magnitude while vectors have both magnitude and direction. Examples of scalars include speed, distance, and temperature. Vectors include velocity, acceleration, force, and displacement.

Since vectors include directional components, they require special mathematical operations compared to scalars. Vector addition involves placing vectors tip-to-tail and summing them to find the resultant vector. This resulting vector will point in a new direction depending on the relative orientation of the original vectors.

Vectors can also be resolved or broken down into perpendicular components. For example, a vector angled 45 degrees from the x and y axes can be resolved into x and y components using trigonometry. The x component equals the vector’s magnitude multiplied by the cosine of the angle, while the y component equals the magnitude multiplied by the sine of the angle.

Understanding the vector nature of motion allows various motions to be combined and analyzed. Vectors enable viewing motion in terms of directional components rather than just magnitudes. This vector perspective underlies many advanced concepts and calculations in physics and engineering.

Projectile Motion

Projectile motion refers to the motion of an object that has been launched or projected into the air, while only being subjected to the force of gravity. Some key aspects of projectile motion include:

Horizontal and Vertical Components: The motion of a projectile can be broken down into horizontal and vertical components. The vertical component is affected by gravity, causing the projectile to accelerate downwards at 9.8 m/s2. However, the horizontal component remains unaffected by gravity, and the projectile will continue moving at a constant horizontal velocity.

Applications: Understanding projectile motion is useful for analyzing the trajectory of objects like balls, rockets or arrows. It allows factors like launch angle and initial velocity to be tweaked to hit targets accurately. Applications include ballistics, sporting events, launching satellites into orbit and more. The equations of projectile motion can calculate values like maximum height, range and time of flight.

Circular Motion

Circular motion is the motion of an object along the circumference of a circle or rotation along a circular path. Some key concepts related to circular motion are:

Centripetal Force

Centripetal force is the net force acting on an object that causes it to follow a curved path. It is directed inward toward the center of the circular path. Common examples are the force exerted on a car by the road on a curved track, or the force acting on a roller coaster car going through a loop. According to Newton’s second law, the centripetal force Fc is given by:

Fc = m * v^2 / r

Where m is the mass of the object, v is its velocity, and r is the radius of the circular path.

Centripetal Acceleration

Since centripetal force causes a change in velocity along the curved path, it results in an acceleration known as centripetal acceleration. It is the acceleration experienced by an object moving in a circular path and directed toward the center. The magnitude of centripetal acceleration ac is given by:

ac = v^2 / r

Centripetal acceleration is always perpendicular to the velocity of the object.

Applications

Circular motion concepts like centripetal force and acceleration have many applications in physics and engineering. Some examples include:

• Rotation of satellites, planets, and celestial bodies
• Banked curves and tilted roads for improved traction
• Centrifuges for separation of mixtures
• Roller coasters, merry-go-rounds, and other circular amusement rides

Understanding the underlying physics allows designing safe and enjoyable rides and other circular motion systems.