What Is Electric Power Formula?
Electric power is an essential concept in electrical engineering and physics. It characterizes the rate at which electrical energy is transferred by an electric circuit. Understanding the formula for electric power provides insight into managing power transfer and losses in electrical systems and components.
Knowing how to calculate electric power is important for designing, operating, and analyzing electrical devices and systems. The power of a circuit determines the rate at which it consumes energy. High power usage leads to higher energy costs, heat dissipation challenges, and greater infrastructure requirements.
This article will provide a comprehensive overview of electric power. We will start with defining power and examining the standard power equation. We will then discuss the factors that affect power and calculations for both DC and AC circuits. Applications of power formulas and ratings will also be covered. By the end, you will have a solid understanding of how to calculate and apply electric power in electrical engineering contexts.
What is Electric Power?
Electric power is the rate at which electric energy is transferred by an electric circuit. It is the amount of energy consumed per unit time. The standard unit of electric power is the watt (W).
Power should not be confused with energy. Energy is measured in joules and is the ability to do work. Power is the rate at which energy is consumed or generated. Even though power and energy are related, they are completely separate concepts.
For example, a light bulb can use 100 joules of energy over a period of 10 seconds, so it consumes energy at a rate of 10 joules per second. The power rating of the light bulb is 10 watts.
In summary, electric power refers to the delivery of energy per unit time and is measured in watts. It describes the rate at which electrical energy is consumed or generated in an electric circuit.
Electric Power Formula
The formula for electric power P is:
P = IV
Where:
- P is power in watts (W)
- I is current in amps (A)
- V is voltage in volts (V)
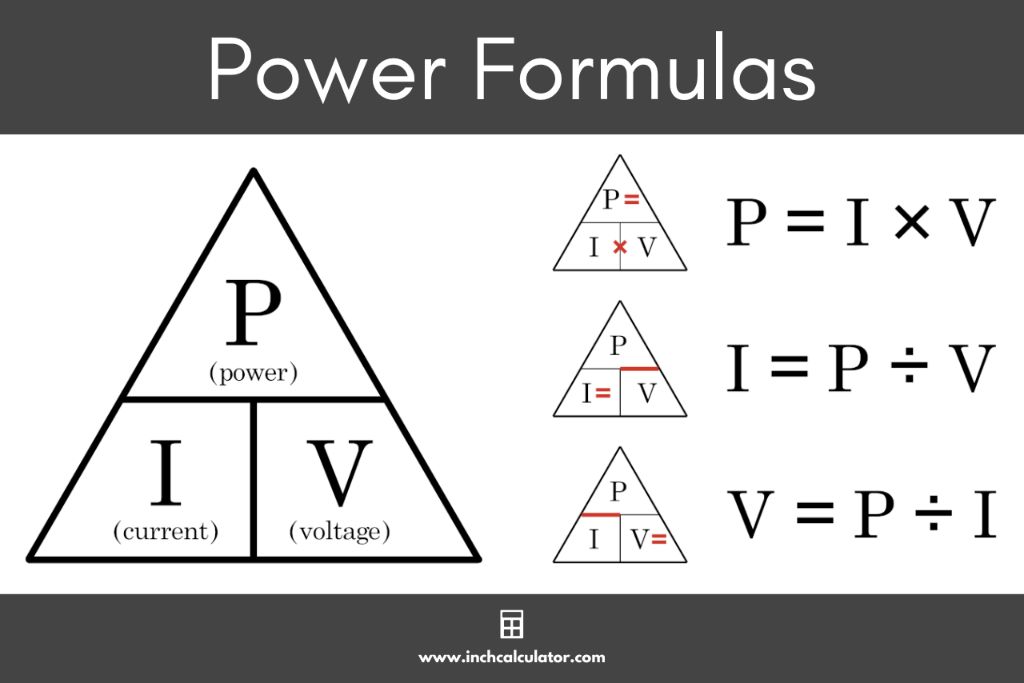
Power represents the rate at which electrical energy is transferred by an electric circuit. It is the product of current and voltage.
Current (I) is the flow of electric charge in a circuit. It is measured in amperes.
Voltage (V) is the difference in electric potential between two points in a circuit. It is measured in volts.
Current, voltage, and resistance are related by Ohm’s Law:
V = IR
Where R is the resistance in ohms.
So according to Ohm’s Law, increasing voltage or current will increase power. Power is also proportional to the square of current based on the power formula.
Factors Affecting Power
There are three key factors that affect electric power according to the power formula – voltage, current, and resistance.
Voltage is the difference in electric potential between two points in a circuit, measured in volts. Current is the rate at which electric charge flows past a point in a circuit, measured in amperes. Resistance is a measure of the opposition to current flow in a circuit component or device, measured in ohms.
Using Ohm’s law, we can see the relationships between voltage, current, and resistance:
Voltage = Current x Resistance
So for a given resistance, increasing voltage will cause current to increase. And for a given voltage, increasing resistance will cause current to decrease. Power is proportional to both voltage and current based on the power formula.
Therefore, increasing voltage in a circuit will increase power. Increasing resistance decreases current, which in turn decreases power. The power dissipated in a resistor is maximum when the resistance equals the source voltage divided by the source current.
Power in DC Circuits
The basic formula for electric power also applies to direct current (DC) circuits:
P = VI
Where:
- P is power in watts (W)
- V is voltage in volts (V)
- I is current in amps (A)
For example, consider a simple DC circuit with:
- Voltage (V) = 12 V
- Current (I) = 3 A
Applying the power formula:
P = VI = (12 V)(3 A) = 36 W
So the power dissipated or consumed in this circuit is 36 watts.
As another example, suppose we have a DC circuit with:
- Voltage (V) = 24 V
- Current (I) = 0.5 A
The power would be:
P = VI = (24 V)(0.5 A) = 12 W
So the power in this circuit is 12 watts.
This demonstrates that power in a DC circuit depends on both current and voltage based on the simple power formula P = VI.
Power in AC Circuits
Power calculations in AC circuits are a bit more complex than in DC circuits because AC voltage and current are constantly varying sinusoidal waves. Since power is voltage multiplied by current, both the voltage and current need to be represented in a way that allows us to calculate their product at any given instant.
To calculate real power in an AC circuit, we use the root-mean-square (RMS) values of voltage and current. RMS represents the equivalent DC voltage or current that would produce the same average power over one cycle. For a sinusoidal wave, the RMS value is simply the peak value divided by the square root of 2.
Therefore, the formula for calculating power in an AC circuit is:
P = VRMS x IRMS
Where:
- P is power in Watts
- VRMS is RMS voltage
- IRMS is RMS current
By using RMS values for voltage and current, we can accurately calculate real power consumption in AC circuits using a simple equation – no need for complex calculus integrals. This makes power calculations for AC just as straightforward as for DC in most cases.
Measuring Power
Power can be measured in electric circuits using a combination of ammeters, voltmeters, and specialized power measurement devices:
Using a Voltmeter and Ammeter
By measuring current and voltage, power can be calculated using the power formula P=IV. An ammeter measures current and a voltmeter measures voltage. Multiplying the voltmeter and ammeter readings together gives power in watts.
Power Meters and Wattmeters
Specialized power measurement devices called wattmeters are also available. These measure both current and voltage, then use the values to directly calculate and display power readings in watts. No manual calculation is required. Power meters operate on the same principle but display the value on a remote readout.
Wattmeters and power meters enable direct and convenient measurement of electric power. However, using separate ammeters and voltmeters provides greater flexibility since current and voltage can also be measured independently.
Applications
The electric power formula is widely used in electrical engineering and energy calculations. Some key applications include:
- Determining the power rating requirements for electrical devices and systems
- Calculating power losses in transmission lines
- Sizing wires, cables, transformers, and other components to safely handle expected power levels
- Understanding electricity usage and energy consumption of homes, buildings, and industrial facilities
- Predicting electricity demand on power grids and planning new power generation accordingly
- Designing and testing batteries, generators, solar panels, motors, and other electrical equipment
The ability to accurately quantify electric power is crucial for electrical engineers, technicians, system operators, and facility managers. Proper application of the power formula helps ensure electrical systems and components run safely, efficiently, and economically.
Since power is the rate of energy consumption or production, the formula also serves a key role in efforts to conserve electricity, promote efficiency, and transition to renewable energy sources.
Power Ratings
Maximum power rating refers to the highest power level in Watts that an electrical device is designed to handle safely and effectively. Exceeding the maximum power rating can lead to overheating, damage, or even fires and electrocution. Respecting a device’s power rating is crucial for safe operation.
Understanding and paying attention to power ratings matters for several reasons:
- Safety – Exceeding the power rating risks dangerous overheating and damage.
- Performance – Operating a device below its rated power allows it to perform as designed.
- Efficiency – Devices are engineered for optimal efficiency around their rated power level.
- Lifespan – Using appropriate power levels extends a device’s lifespan by avoiding unnecessary strain.
- Regulations – Many jurisdictions regulate device power for safety reasons.
Checking and adhering to power ratings protects users, optimizes device operation, reduces fire hazards, and ensures compliance with local regulations.
Conclusion
In summary, electric power (P) is defined as the electric energy consumed per unit time that is transmitted to a load. It can be calculated using the formula P = VI, where V is voltage and I is current. Understanding electric power is crucial for properly rating electrical devices and transmission systems to ensure safe and reliable operation.
Calculating power allows electrical engineers and technicians to size wires, transformers, and other components to accommodate expected loads without overheating or other issues. It also aids in evaluating the efficiency of systems by comparing input and output power. With both AC and DC power, the principles are similar but calculations must account for differences in how voltage and current behave between the two.
Knowing how to accurately measure and compute electric power provides a foundational understanding for working with almost any electrical or electronic system. Whether it’s wiring a house, designing an electric vehicle charging station, or developing a large-scale power plant, the ability to work with electric power is essential.