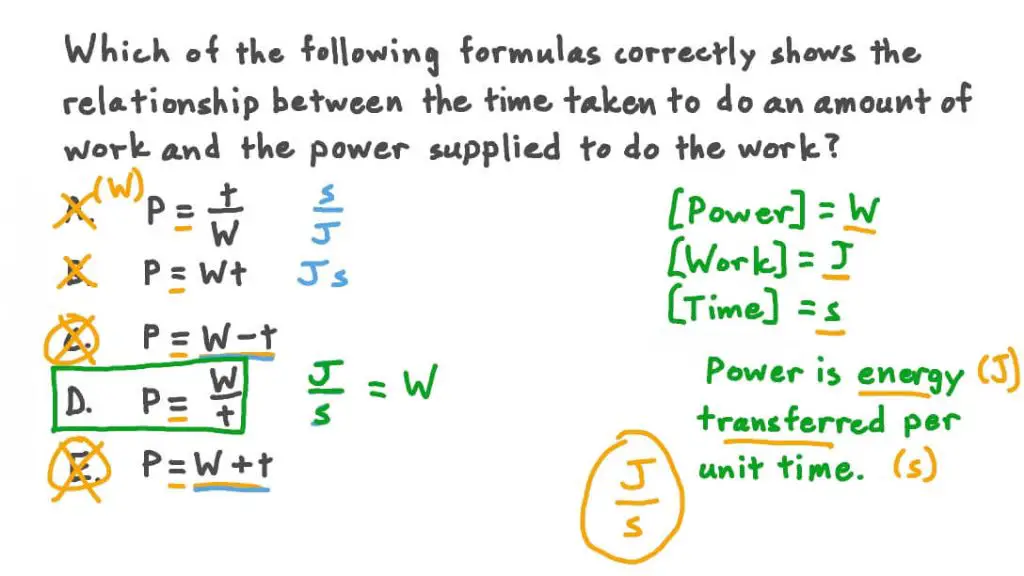
What Are The 3 Formulas For Power?

Power is the rate at which energy is transferred, used, or transformed over a specific period of time. Understanding power is important in many scientific and engineering fields like physics, electronics, mechanics, and energy systems. Knowing how to calculate power allows us to determine the rate at which devices use energy, size components properly, analyze the capabilities of power sources, and understand efficiencies. The basic unit of power is the watt, defined as 1 joule per second. There are several common formulas for calculating power based on different parameters.

Table of Contents

Physics Power Formula

The most fundamental power formula is derived from physics. It relates power (P) to current (I) and voltage (V). The formula is:

P = IV

Where:

P is power in watts (W)
I is current in amps (A)

V is voltage in volts (V)

This formula shows that power is equal to the product of current and voltage. Intuitively, this makes sense, since increasing either the current or voltage in a circuit will lead to an increase in power. This relationship is used extensively in electrical engineering and physics when analyzing electric circuits.

Electrical Power Formula

The formula for electrical power is:

P = VI

Where:

P is power in watts (W)

V is voltage in volts (V)
I is current in amps (A)

This formula shows that power (P) equals voltage (V) multiplied by current (I). Intuitively, this makes sense – more voltage or more current will result in more power. Electrical devices operate by consuming power. The rate at which they consume power is measured in watts.

For example, if a device operates on 120V and draws 2A of current, you can calculate the power using the formula:

P = VI = (120V)(2A) = 240W

So this device consumes electrical power at a rate of 240 watts.

The electrical power formula is useful for calculating the power consumption of electrical devices. When multiplied together, voltage and current give the rate of energy transfer, which is power.

Mechanical Power Formula

The mechanical power formula is used to calculate the rate at which work is done or energy is transferred in mechanical systems. The formula is:

P = Fv

Where:

P is power in watts (W)
F is force in newtons (N)

v is velocity in meters per second (m/s)

This formula shows that power is equal to the product of force and velocity. So the more force exerted or the greater the velocity, the more mechanical power is produced. This relationship makes sense intuitively – the harder you push and the faster something moves, the more work is being done per unit time.

Some examples of using this mechanical power equation are calculating power output of a motor, engine power of a vehicle, or power during weightlifting exercises. The force and velocity values are inserted into the formula to determine the rate at which the system is doing work.

Relationships Between the Formulas

The physics, electrical, and mechanical power formulas are all related in that they describe the rate at which energy is transferred or work is done. However, each one applies to a different context.

The physics power formula P = E/t relates power to the rate of change of energy E over time t. This is the most general formula that can apply to any form of power. The electrical power formula P = IV relates power to current I and voltage V in an electrical circuit. The mechanical power formula P = Fv relates power to force F and velocity v for mechanical systems.

While the physics power formula can describe electrical and mechanical power using the appropriate energy and time variables, the electrical and mechanical formulas are more specific to their respective contexts. The electrical formula uses current and voltage, which only apply to electrical systems. The mechanical formula uses force and velocity, which only apply to mechanical systems.

So in summary, the physics power formula is the most general, while the electrical and mechanical formulas describe power in more specific contexts by using variables unique to electricity and mechanics. But ultimately they all describe the same underlying relationship between power and the rate of energy transfer.

Using the Formulas

To understand when and how to use each of the main power formulas, let’s walk through some examples.

To use the physics power formula, you may need to calculate the power output of an engine. If you know the amount of work (W) done is 250 Joules, and the time (t) taken is 50 seconds, you can calculate:

Power = Work / Time
Power = 250 J / 50 s

Power = 5 W

For the electrical power formula, you may want to determine the power consumption of an appliance. If you know the voltage (V) is 120 volts, and the current (I) is 5 amps, you can determine:

Power = Voltage x Current
Power = 120 V x 5 A
Power = 600 W

Finally, the mechanical power formula is useful for calculating power in rotational mechanical systems. If a motor has a torque (τ) of 300 Nm and an angular velocity (ω) of 10 rad/s, then:

Power = Torque x Angular Velocity

Power = 300 Nm x 10 rad/s
Power = 3000 W

As you can see, each formula allows you to calculate power in different systems, given the relevant variables. The key is understanding what data you have, and choosing the right formula.

Power Units

There are a few common units used to measure power:

Watts (W) – Watts are the standard SI unit for power. It measures the rate of energy conversion or transfer. For example, a 100W lightbulb converts 100 joules of electrical energy per second into light and heat energy.
Kilowatts (kW) – Kilowatts are equal to 1000 Watts. Kilowatts are commonly used to measure the power output of electrical generators and motors.

Megawatts (MW) – Megawatts are equal to one million Watts. Megawatts are used to measure the generating capacity of power plants and the consumption of large facilities.
Horsepower (hp) – Horsepower is a non-SI unit commonly used to rate the power of combustion engines like those in cars and trucks. One horsepower equals about 746 Watts.
BTUs per hour – BTU stands for British Thermal Unit, a traditional unit of power often used for HVAC systems and appliances. One BTU per hour is approximately 0.2931 Watts.

Knowing the relationships between these units allows you to convert power measurements between Watts, horsepower, BTUs, etc. as needed.

When to Use Each Formula

The choice of which power formula to use depends on the information you have available and the type of power you are calculating.

Use the physics power formula (P=W/t) when you know the amount of work being done and the time taken. This can calculate power in any situation that involves work and time.

Use the electrical power formula (P=VI) to find power in electrical circuits and devices. This formula uses voltage and current, which are easily measured properties of electricity.

Use the mechanical power formula (P=τω) when dealing with rotational mechanical systems like motors, turbines, and gears. This formula uses torque and angular velocity.

In summary:

Physics power formula (P=W/t) – general power situations involving work and time
Electrical power formula (P=VI) – electrical circuits and devices
Mechanical power formula (P=τω) – rotational mechanical systems

Knowing when to apply each formula allows you to calculate power in any scenario you encounter.

Other Power Formulas

There are a few other lesser-known power formulas worth mentioning briefly:

Wind Power – The power available from wind can be calculated using P = 0.5*ρ*A*v^3 where ρ is the air density, A is the area swept by the turbine blades, and v is the wind speed.

Power in AC Circuits – For AC circuits, power is calculated as P = Vrms * Irms * cosθ where Vrms and Irms are the root-mean-square voltage and current values and cosθ is the power factor.

Hydraulic Power – The power of hydraulic equipment can be found using P = p*Q where p is the pressure and Q is the flow rate.

Power of a Spring – The power released when a spring recoils is calculated as P = 0.5*k*x^2*v where k is the spring constant, x is the displacement, and v is the velocity.

While not used as widely as the main power formulas, these can be handy for specialized engineering applications.

Conclusion

Looking back over the three formulas for power, we remember that power reflects how fast work is done or energy is transferred. The physics power formula shows that power is equal to work divided by time. For electrical circuits, power is equal to voltage multiplied by current. And for mechanical systems, power comes from force multiplied by velocity.

The key takeaways around these formulas are:

They each reveal a different relationship that generates power in different contexts.
Units must be consistent within each formula.
Power is measured in watts in the SI system, reflecting transfers of joules per second.

To properly calculate power, you need to identify the system you’re working with and select the suitable formula.

With an understanding of these core power formulas and how to apply them, you can determine power outputs and requirements in physics, electrical, and mechanical systems.