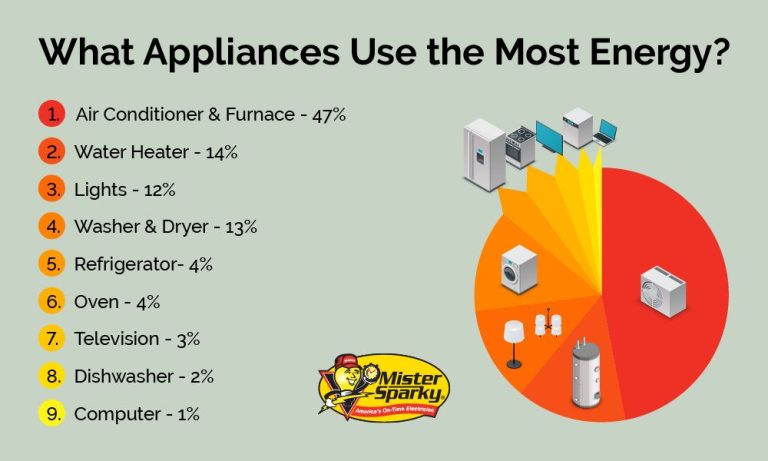
Does Kinetic Energy Increase As The Mass Of An Object Increases?

Kinetic energy is the energy an object has due to its motion. The faster an object moves, the more kinetic energy it has. Kinetic energy is directly proportional to the mass and velocity of an object.

The formula for kinetic energy is:

Kinetic Energy = (1/2) x Mass x Velocity^2

Where:

• Mass is measured in kilograms (kg)
• Velocity is measured in meters per second (m/s)

As seen in the formula, kinetic energy increases proportionally with mass. Therefore, as the mass of an object increases, its kinetic energy will also increase assuming its velocity remains constant.

Kinetic Energy Formula

The formula for kinetic energy is:

KE = 1/2mv²

Where:

• KE = Kinetic Energy (in Joules)
• m = Mass (in kilograms)
• v = Velocity (in meters per second)

This formula shows that the kinetic energy of an object depends on two variables – its mass and its velocity. The mass (m) is a measure of how much matter the object contains. The velocity (v) is a measure of the object’s speed in a particular direction.

Let’s break down what each part of the formula means:

• The 1/2 is a constant that is derived from calculus and the physics of motion.
• m refers to the object’s mass. The more massive an object is, the more kinetic energy it will have at a given velocity.
• v refers to the object’s velocity squared. Squaring the velocity in the formula amplifies the effect that velocity has on kinetic energy.

So in summary, the kinetic energy formula shows us that the kinetic energy of an object depends on both its mass and its velocity squared.

Mass in the Kinetic Energy Formula

The formula for kinetic energy is K.E. = 1/2 mv^2, where m is the mass of the object and v is its velocity. This shows that kinetic energy is directly proportional to the mass – as mass (m) increases, kinetic energy increases proportionally. Specifically, if the mass is doubled, the kinetic energy will also double. This makes sense intuitively, since heavier objects require more effort to accelerate and decelerate compared to lighter objects. For example, a truck has much higher kinetic energy at 60mph compared to a small car at 60mph due to its greater mass.

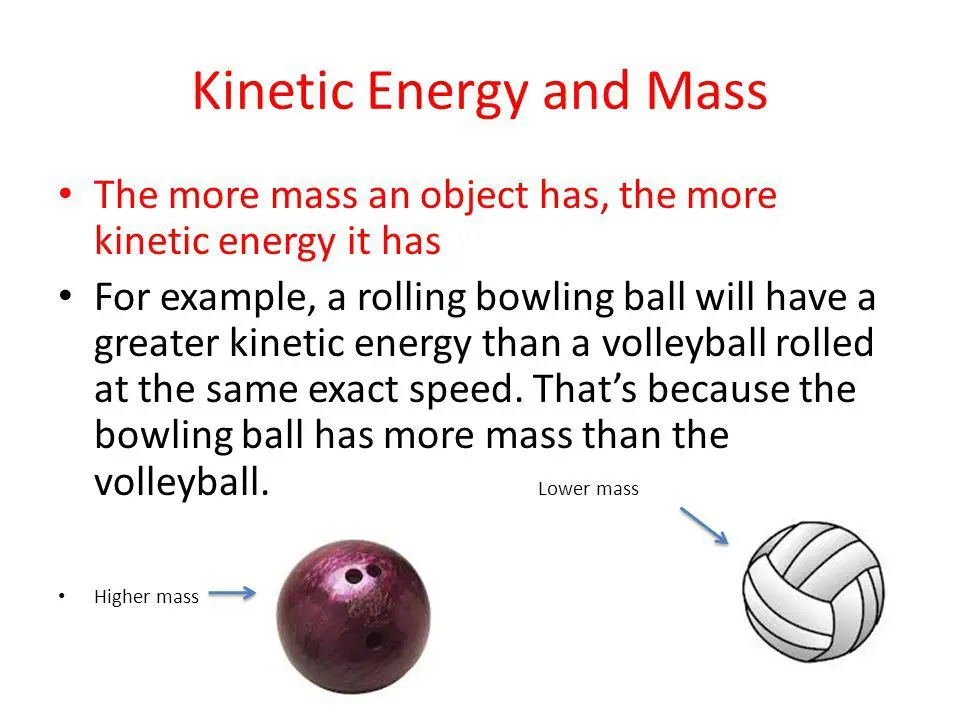
The mass term in the kinetic energy formula accounts for the fact that heavier objects in motion have more energy than lighter objects moving at the same speed. This relationship is linear – doubling the mass doubles the kinetic energy, tripling the mass triples it, etc. In physical terms, more massive objects experience greater inertia, requiring more force to accelerate or decelerate their motion.

Intuitive Explanation

Intuitively, it makes sense that the kinetic energy of an object increases as the mass increases. Think about the difference between a bicycle and a truck traveling at the same speed and colliding with something. The truck has much more mass than the bicycle, so it’s going to have a lot more kinetic energy and cause much more damage upon impact. The heavier truck takes more effort to get moving and takes more effort to stop once it’s moving. This is because kinetic energy is proportional to mass – double the mass and you double the kinetic energy. The massive truck packs a punch due to all that kinetic energy it has from its large mass.

Kinetic energy can be thought of as the “bigness” or “power” of an object in motion. The more massive an object is, the more kinetic energy it will have at the same speed. This intuitive understanding helps explain why heavier objects like trucks or freight trains cause so much more damage than lighter objects when crashing, even if both are traveling at the same velocity. The physics concept of kinetic energy captures this idea that mass and motion together create powerful forces.

Kinetic Energy of Objects with Different Masses

To demonstrate how kinetic energy increases with mass, let’s look at some examples of objects with different masses and calculate their kinetic energies:

Object 1: Baseball (mass = 0.145 kg)
Velocity = 30 m/s

Kinetic Energy = 0.5 x 0.145 kg x (30 m/s)2 = 65.25 J

Object 2: Bowling ball (mass = 7.3 kg)

Velocity = 30 m/s
Kinetic Energy = 0.5 x 7.3 kg x (30 m/s)2 = 32,850 J

As you can see from the calculations, even though both objects have the same velocity, the bowling ball has a much greater kinetic energy than the baseball, because of its larger mass. The bowling ball’s kinetic energy is over 500 times greater than the baseball’s!

This demonstrates that as an object’s mass increases, its kinetic energy will also increase exponentially, assuming its velocity stays constant. The kinetic energy formula shows the direct relationship between an object’s mass and its kinetic energy due to the mass term being multiplied by the velocity squared.

Exceptions and Special Cases

There are a few exceptions where mass does not affect kinetic energy in the normal way. These primarily relate to massless particles like photons.

Photons are particles of light that have no mass. However, they do have momentum and energy. The kinetic energy of a photon is calculated using a different formula that does not depend on mass:

Ek = pc

Where p is the photon’s momentum and c is the speed of light in a vacuum. Since photons have no mass, their kinetic energy only depends on their momentum and the speed of light, not their mass.

Another potential exception relates to objects moving at relativistic speeds near the speed of light. At these speeds, the effects of special relativity come into play. The relativistic kinetic energy formula includes a dependence on the Lorentz factor, which takes into account time dilation and length contraction effects.

So in summary, for normal objects moving at everyday speeds, increased mass leads to increased kinetic energy. But massless particles like photons and objects moving at very high relativistic speeds are exceptions where the kinetic energy does not have the normal dependence on mass.

Applications and Examples

Kinetic energy’s relationship with mass has important practical applications and real-world examples. Here are a few:

• In car crashes, a heavier vehicle carries more kinetic energy, resulting in more damage in a collision. This is why large SUVs and trucks can be dangerous on the road.

• In sports like boxing and football, a heavier athlete packs more of a punch. Their kinetic energy transfers into a bigger impact when they collide with an opponent.

• Rockets need huge amounts of fuel to accelerate their massive bodies to high speeds. More mass makes them harder to get moving.

• Wrecking balls demolish buildings because of their great mass swung at high speeds. More mass concentrates the kinetic energy in a small point of impact.

• Meteorites do more damage if they have more mass. A small meteor burns up, while a large one like the dinosaur killer carries cataclysmic kinetic energy.

In all these examples, more mass means more concentrated kinetic energy, creating a bigger effect when that energy transfers to another object.

Frequently Asked Questions

Here are some common questions about the relationship between an object’s kinetic energy and its mass:

Does kinetic energy always increase with mass?

Yes, assuming all other variables like velocity stay constant, kinetic energy will always increase proportionally with mass. The kinetic energy equation shows that mass is directly proportional to kinetic energy.

Does this relationship apply at relativistic speeds?

At speeds approaching the speed of light, the kinetic energy equation needs to be adjusted to account for relativistic effects. The classic kinetic energy equation only applies accurately at low, non-relativistic speeds. At very high velocities, kinetic energy increases at a lower rate as mass increases compared to the non-relativistic case.

What if the object’s velocity changes?

If the velocity of the object changes while its mass stays constant, then its kinetic energy will change. Kinetic energy depends on both mass and velocity. So if velocity increases, kinetic energy will increase even if mass stays the same. The relationship between mass and kinetic energy only holds true if velocity remains unchanged.

Does this apply to photons?

Photons are massless particles, but they still have momentum and kinetic energy proportional to their frequency. So for photons, kinetic energy does not depend on mass, since they have no mass. The standard kinetic energy formula does not apply to photons.

Summary

In summary, kinetic energy does increase as the mass of an object increases, assuming the velocity remains constant. This is because kinetic energy is calculated as 1/2 x mass x velocity squared. Therefore, if mass increases while velocity stays the same, the kinetic energy will increase in direct proportion to the increase in mass.

To recap the key points:

• Kinetic energy is defined by the formula KE = 1/2 x m x v^2, where m is mass and v is velocity.
• Mass has a direct relationship with kinetic energy – as mass increases, kinetic energy increases.
• This is because mass is multiplied directly by velocity squared in the formula.
• The velocity term stays constant if the speed of the object is unchanged.
• Therefore, increasing the mass will increase the kinetic energy in direct proportion.

In conclusion, the kinetic energy of an object does reliably increase as the mass increases, assuming its velocity remains unchanged. This demonstrates the direct relationship between mass and kinetic energy.

References

[1] University Physics, OpenStax, https://openstax.org/details/books/university-physics-volume-1

[2] The Physics Classroom, http://www.physicsclassroom.com/class/energy/Lesson-1/Kinetic-Energy

[3] HyperPhysics, Georgia State University, http://hyperphysics.phy-astr.gsu.edu/hbase/ke.html

[4] NASA, https://www.grc.nasa.gov/www/k-12/airplane/ke.html

[5] Khan Academy, https://www.khanacademy.org/science/physics/work-and-energy/work-and-energy-tutorial/a/what-is-kinetic-energy